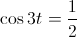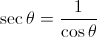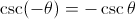The two main purposes of these formulas are finding exact values of other trig functions and simplifying expressions to find other identities
Sum and Difference Formulas for sin, cos, and tan:
 Example 1:
Example 1: Find the exact value of cos 75°
cos75°=cos(30°+45°)
=cos 30° cos 45° - sin 30° sin 45°

You can check your answer by plugging it into your calculator. cos 75° is about 0.259, so our answer is correct!
Example 2: Find the exact value of